Friday, 21 July 2017
Halo simulation using a single ice crystal population
Halo simulation aims at finding those ice crystal shape and orientation parameters that best reproduce observed characteristics in a given display. Publicly available simulation software provide a great deal of flexibility by allowing users to include several separate crystal populations and by giving them free hands to set population-specific crystal shape and orientation parameters as they see fit. For example, simulating the 22nd September 2012 display (shown above) would require including at least five crystal populations. These are necessary as the observed halos associate with all five principal types of crystal orientation (random, plate, column, Parry, and Lowitz orientations), and each population accommodates only one orientation type. As each population needs its own shape and orientation parameters, the total number of input parameters is almost impractical.
I have recently entertained myself with the idea of introducing a mutual dependence between the orientation and shape parameters. This could reduce some of the complexity currently associated with the simulation. In such framework, even complicated-looking displays can be reasonably well simulated using only a handful of user-specified input parameters. Furthermore, the idea of only one crystal population in one halo-making cloud is conceptually appealing.
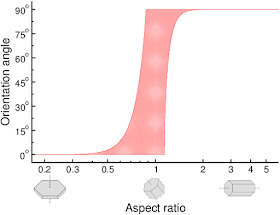
To illustrate the approach I've been thinking of, the figure above shows a possible orientation-shape dependency. Let's consider, for now, the aspect ratio as the only measure of crystal shape. The y-axis representing the angle of crystal's axis with local vertical, perfect plate and column orientations correspond to values 0° and 90°, respectively. We assume a Gaussian distribution such that 95% of crystals (at each aspect ratio) go within the shaded region. In the regime of equidimensional crystals in the middle, the orientation angle takes all values between 0° and 90°. Otherwise, it is constrained around either 0° or 90°, depending on whether the shape is more like a plate or rather like a column. The constraint is particularly strict in the extremes at far left and far right, indicating that perfect orientations are most probable to occur with the thinnest plates and longest columns.
I have produced the all-sky simulations below by running a home-made simulation program and assuming the orientation-shape dependency exactly as laid out above. Each simulation uses only one crystal population, and the six simulations differ in only two input parameters that control the distribution of crystal aspect ratio. At the top, the distribution is set narrow such that the crystals are either (a) all plates, (b) all equidimensionals, or (c) all columns. In panels (d)-(f), the distribution is made gradually wider around the equidimensional mean. Clearly, changing just two input parameters is enough to allow reproducing a variety of displays.
To simulate occurrences of Parry and Lowitz arcs, we need to introduce another dependency to constrain crystal's rotation about its axis. In my trials, I have assumed that the rotation averages to that required in the Parry orientation, but the spread around the mean rotation depends on crystal base shape (see the figure below for illustration). Only crystals with a considerable tendency towards either triangular or tabular habit are assigned with spread small enough to allow Parry arcs to form. For conventional hexagons and other possible base shapes, we set the spread large to make the distribution of rotation angles essentially uniform.
Now, using the two dependences as laid out above and running my home-made program, panels (a)-(c) below illustrate the effect of varying the distribution of column crystal base shapes alone. Four input parameters are used to control these variations. In panel (a), all crystals are nearly regular hexagons in their bases. Halos attributed to the column orientation are well reproduced, and no signs of Parry arcs are seen. In panel (c), crystals are constrained to the tabular base shape. This scenario produces only halos associated with the Parry orientation. Panel (b) is for the case where the base shape varies considerably, such that halos associated with both column and Parry orientations are represented.
To get not just Parry but also Lowitz arcs, one needs to take benefit from the two types of dependence at once. This is attempted in panels (d)-(f) for three different solar elevations. Although the all-sky projection makes direct comparison slightly complicated, I'd say the similarity of panels (d) and (e) with the appearance of the 22nd September display is remarkable.
While assuming the same dependencies to hold for the behaviour of orientation and rotation angles as a function of crystal shape, all variations in the simulations shown here were produced by changing just seven input parameters. One goes for the solar elevation, and the remaining six were used to characterize crystal shapes.




This is a really fascinating hypothesis, Reima. I hope that those in the community with more technical understanding than I will be able to investigate and develop this idea further. What I have been wondering is what impact if any would this have on the user interface of simulation software. Would you envisage it being radically streamlined and stripped down compared say to HaloPoint as it currently exists? Could you imagine software evolving to the point where you basically have two or three interdependent sliders and a small number of check boxes for variable parameters? Also could this possibly be ported to a mobile app making use of gps and time to automatically record solar/lunar elevation? In live view, you could also incorporate br processing then transparently overlay the resultant sim. Absolutely loads of potential for features.....!
ReplyDeleteHi Reima
ReplyDeletegreat work as usual! i think what we miss for simulation is multiphysics software that would be able to tell from a polygonal shape definition (ice crystal), what is the actual orientation taken in freefall.
what you did is introduce manually a general dependency between orientation and shape. next major step would be definitely to validate your approach with data based on a multiphysics software. this would be great help to validate many points, like the way a 34° prism orient (Moilanen arc).
based on some simple approach like relative position of center of gravity vs rotation torque position when a pressure is applied to the crystal face, i get that 34° prismatic crystal should fall pointing up while half pyramidal crystals (conical instead of prismatic) should fall pointing down. i tried at some point some multiphysics software but could not get useful stuff. that would be a real breakthrough in simulation and explain some weird stuff we see if we could just send crystal shape (and size to accomodate reynolds number/brownian motion) to software and get the directly the statistical orientation parameter... maybe you know some tools that could do that?
Thanks for the comments, Alec and Nicolas. I had not really considered from the user interface point of view, as my intention was not to replace existing tools. Rather, I was hoping to improve our general understanding on halos and why they show up the way they do. We have seen how halos that associate with different orientation types are all at their purest at the same time. It takes fewer assumptions to explain such behaviour as coming from variations in a single population than from a combination of several independent populations. Keeping things simple, that’s the idea.
ReplyDeleteBut yes, I can imagine a user interface where you specify one set of parameters to determine the exact form for the dependence of orientation and rotation angles on crystal shape (and possibly size), and another set to describe crystal shapes (ideally the former would be more universal than the latter).
About the multiphysics software Nicolas is suggesting, I have not much to contribute. I am not familiar with that sort of stuff. Sounds like a useful concept when dealing with certain exoticish displays, but might be a bit overkill when it comes to ordinary cirrus displays witnessed by ordinary people. If that’s what’s needed to simulate the Moilanen arc, fine for me. But in a more general setting, I don’t think it’s just about the free-fall orientation we want to get right: we also need to quantify the amount of spread around that. Otherwise we need to continue allocating one population for circular halos only.
when we do the usual simulations, we indeed do "manually" (with too parameters) what is done automatically with your approach. we input plate crystals in plate orientation, then we input crystals which have height/width ratio ~1 in random orientation, then we input long crystals in column orientation.
ReplyDeletethis is discrete approximation, while your approach is definitely better as it gives a continuous approximation.
still, you set manually the orientation behavior vs shape, like we do. just that it is continuous rather than discrete.
next step is really to remove this manual link between orientation and shape, which we do by habit, and unverified link about what we assume to be the correct link. so it suffers the same limitation that it could be wrong on some shape and on the limits between different behavior.
multi physics software should allow to get all the distribution of possible orientations by giving the stability of the each individual orientation. i would be really interested in seeing someone with knowledge on those software giving it a try...